Roman Numbers: Chart, Rules, Conversation, Questions

Have you ever puzzled about the enigmatic symbols on clock faces or in movie credits? Do you find it difficult to comprehend what they are saying? You are not alone! Roman numerals can be difficult to understand at first. But do not be afraid! In this post, we’ll uncover the mystery of Roman numerals in a simple and easy-to-understand approach, including roman numbers chart, rules, conversation between Numbers and Roman Numerals, and Practice Questions on Roman Numerals.

The most common roman numerals that are presently used to represent the basic numbers are given in the table below. 1 5 10 50 100 500 1000 Roman Numerals Chart (1 to 1000) Below is the chart for roman numerals that shows the roman numerals from 1 to 1000.

Roman numerals are often used for hour marks on clock faces, showcasing their practical application beyond just counting and representation of numbers. This makes them a great topic to explore in math class, as they combine history and mathematics seamlessly.

Whether you’re struggling with history homework or simply curious about ancient symbols, we’ve got you covered. Let’s begin the journey to decipher Roman numerals and uncover their mysteries without breaking a sweat! Join us to learn math in a fun and engaging way!

Part 1. What are Roman Numerals?

Roman numerals are a numeral system that originated in ancient Rome. Roman numbers, unlike the Arabic digits we use today (1, 2, 3, etc.), are made out of Latin letters from the alphabet, specifically the letters I, V, X, L, C, D, and M. Each letter has a numerical value given to it, as detailed in many educational resources, including those from Cambridge University Press.

• I = 1
• V = 5
• X = 10
• L = 50
• C = 100
• D = 500
• M = 1000

Formation of Numbers:

Roman numerals are formed by combining these basic symbols in specific ways. The key rules to remember are:

• Symbols are arranged from left to right in decreasing order of value.
• If a symbol of lower value appears to the left of a symbol of higher value, you subtract the lower value from the higher value.
• If a symbol of lower value appears to the right of a greater value symbol, you add the lower value to the greater value symbol.

For example:

The following are a few examples of how Roman numerals are formed:

• II = 2 (Two I’s)
• IV = 4 (One before five, subtracted from five)
• IX = 9 (One before ten, subtracted from ten)
• XX = 20 (Two tens)
• XL = 40 (Ten before fifty, subtracted from fifty)
• XC = 90 (Ten before hundred, subtracted from hundred)
• CD = 400 (Hundred before five hundred, subtracted from five hundred)
• CM = 900 (Hundred before thousand, subtracted from thousand)

History of Roman Numerals:

Roman numerals, used by the Romans, originated in ancient Rome and were widely employed in daily life, business, and government documents. The system changed throughout time, as different symbols and standards were accepted and perfected.

Roman numerals were the primary numeral system used in Europe for centuries, from the Middle Ages to the Renaissance. They remained popular until the late Middle Ages, when Arabic numerals were widely adopted.

Modern Usage of Roman Numerals:

While Arabic numerals are the standard numerical system used worldwide today, Roman numerals remain significant in a variety of circumstances.

• Clock Faces: Many clock faces continue to utilize Roman numerals to depict the hours.
• Book Chapters: Roman numerals are commonly used to designate the chapters or divisions of a book.
• Page Numbering: Roman numerals play a role in organizing content in books and chapters, enhancing readability and navigation.
• Movie Credits: They are frequently used in film credits to denote the production year or sequel number.
• Numbering Outlines: Roman numerals are occasionally used to number outlines, particularly in formal documents and academic works.

Part 2. Roman Numerals Chart (1 to 1000)

When translating a Roman numeral into an integer value or vice versa, the Roman numerals chart comes rather handy. Roman numerals are used to depict the numbers in the following list. Roman numerals ranging from 1 to 1000 are seen here below: 1, 2, 3,…, 10, 11, 20, 30, 50, 100, 500, 1000, 2000, 3000,… Roman numbers from 1 to 3000 can be written quickly with the chart. The chart helps students learn how to write Roman numbers.

1	I		11	XI		200	CC		1	I
2	II		20	XX		300	CCC		5	V
3	III		30	XXX		400	CD		10	X
4	IV		40	XL		500	D		50	L
5	V		50	L		600	DC		100	C
6	VI		60	LX		700	DCC		500	D
7	VII		70	LXX		800	DCCC		1000	M
8	VIII		80	LXXX		900	CM		2000	MM
9	IX		90	XC		1000	M		3000	MMM
10	X		100	C		1001	MI

Printable Roman Numerals Chart – Download PDF

Roman Numerals 1 to 20	Download PDF
Roman Numerals 1 to 50	Download PDF
Roman Numerals 1 to 100	Download PDF
Roman Numerals 1 to 200	Download PDF
Roman Numerals 1 to 500	Download PDF
Roman Numerals 1 to 1000	Download PDF
Roman Numerals 100 to 1000	Download PDF

Part 3. Rules of Roman Numerals

Understanding how to represent numbers with the roman numeral system requires adhering to precise standards. Let’s look at these guidelines to ensure appropriate representation:

Rule	Description
1	When certain numerals are repeated, the number represented by them is their sum. For example, II = 1 + 1 = 2, XX = 10 + 10 = 20, XXX = 10 + 10 + 10 = 30. The Roman numeral system can represent both base and arbitrary numbers through combinations of symbols.
2	No Roman numerals can come together more than 3 times. For example, we cannot write 40 as XXXX.
3	The letters V, L, and D are not repeated.
4	Only I, X, and C can be used as subtractive numerals. There can be 6 combinations when we subtract: IV = 5 – 1 = 4; IX = 10 – 1 = 9; XL = 50 – 10 = 40; XC = 100 – 10 = 90; CD = 500 – 100 = 400; CM = 1000 – 100 = 900.
5	When a Roman numeral is placed after another Roman numeral of greater value, the result is the sum of the numerals. For example, VIII = 5 + 1 + 1 + 1 = 8, or XV = 10 + 5 = 15.
6	When a Roman numeral is placed before another Roman numeral of greater value, the result is the difference between the numerals. For example, IV = 5 – 1 = 4, XL = 50 – 10 = 40, XC = 100 – 10 = 90.
7	When a Roman numeral of a smaller value is placed between two numerals of greater value, it is subtracted from the numeral on its right. For example, XIV = 10 + (5 – 1) = 14, or XIX = 10 + (10 – 1) = 19.
8	To multiply a number by a factor of 1000, a bar is placed over it.
9	Roman numerals do not follow any place value system.
10	There is no Roman numeral for zero (0).

Rules of Reading Roman Numerals?

Here are some extra guidelines and nuances for understanding Roman numerals. Understanding and using these guidelines will significantly improve your accuracy when reading Roman numerals, ensuring that each numeral is understood in the correct numerical value.

Rule Number	Description
1	Numerals Repeat: A numeral can be repeated up to three times to form the numbers. For example, III = 3 and XXX = 30.
2	Descending Order: Place larger numerals before smaller ones to add them. For example, VI = 6 (5 + 1).
3	Subtractive Notation: Place a smaller numeral before a larger one to subtract the smaller from the larger. For example, IV = 4 (5 – 1).
4	No Repeating V, L, D: The numerals V (5), L (50), and D (500) cannot be repeated.
5	Combination Rules: The correct combinations of numerals must be followed to ensure proper numeral structure. For example, IX = 9, not VIIII.
6	Limited Subtractive Pairs: Only certain pairs are allowed for subtractive combinations: IV (4), IX (9), XL (40), XC (90), CD (400), and CM (900).
7	Maximum Three: A numeral can be repeated a maximum of three times in succession for the same digit. For example, III is 3, but IIII is not valid for 4 (instead, IV is used).
8	Order of Numerals: Numerals must be arranged from highest to lowest from left to right, except in the case of subtractive pairs.
9	Avoiding Four Repeats: The same numeral should not appear four times in succession; this is where subtractive notation comes into play to maintain numeral efficiency.
10	Read from Left to Right: Roman numerals should be read from left to right, adding or subtracting values as dictated by their order and combination.

Rules for Writing Roman Numerals

Understanding how to represent numbers using Roman numerals involves following specific rules. The following guidelines will help you learn how to write Roman numerals accurately. Let’s explore these guidelines to ensure accurate representation:

Rule or Principle	Description
Repetition Rule	Symbols are repeated to represent the value they hold. For instance, II represents 2, XX represents 20, and XXX represents 30.
Limit on Repetition	A symbol can be repeated only up to three times consecutively. For example, XXX denotes 30, and CC signifies 200.
Non-Repeating Symbols	Certain symbols, namely V, L, and D, are never repeated. You won’t see VV or LL, maintaining integrity of the numeral system.
Addition Principle	When a symbol of lesser value appears after a symbol of greater value, their values are added together. For example, VI represents 6 (5 + 1).
Subtraction Principle	When a symbol of lesser value appears before a symbol of greater value, it indicates subtraction. For example, IX represents 9 (10 – 1).
Limitation on Subtraction	The symbols V, L, and D are never subtracted, as they are not positioned before greater value symbols, preventing confusion.
Specific Subtraction Pairs	The symbol I can be subtracted from V and X only, while X can be subtracted from L, C, and M only.

Part 4. Roman Numerals Worksheet

ReWorksheets focusing on Roman numerals develop both reading and writing skills. These sections contain a variety of topics, including word puzzles, number conversions (to and from Roman numerals), and more. For your convenience, certain Roman-numbered worksheet pages are supplied in PDF format.

Printable Roman Numerals Worksheet – Download PDF

Part5. Roman Numerals to Numbers

Roman numerals are used to denote various values in different contexts, such as dates and levels of brightness. To convert Roman numerals to numbers, you must first learn the values of various Roman symbols and then follow a few simple rules. Here’s a fast guide for converting Roman numerals to their matching numbers. Let’s go over the process in detail.

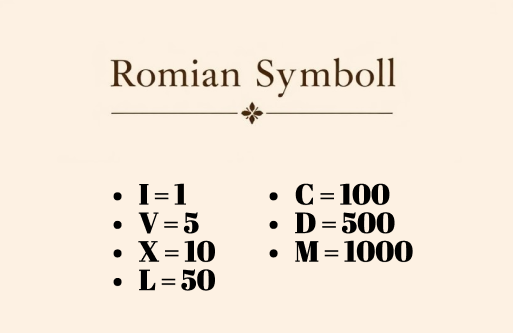
Roman Numeral Symbols

Each Roman numeral symbol represents a specific value:

• I = 1
• V = 5
• X = 10
• L = 50
• C = 100
• D = 500
• M = 1000

Conversion Rules

1. Addition Rule: If a numeral is followed by another of equal or lesser value, the values are added.
   1. Example: VII = 5 + 1 + 1 = 7
   2. Example: LXXX = 50 + 10 + 10 + 10 = 80
2. Subtraction Rule: If a numeral is placed before another of greater value, it is subtracted from the higher value.
   1. Example: IX = 10 – 1 = 9
   2. Example: CM = 1000 – 100 = 900
3. Sequential Rule: Read from left to right, applying the addition or subtraction rule as appropriate.
   1. Example: MCCC = 1000 + 100 + 100 + 100 = 1300
   2. Example: XC = 100 – 10 = 90

Applying the Rules:

To convert a Roman numeral to a number, start from the left and work your way to the right, deciding at each step whether to add or subtract based on the relative values of the numerals. Here are some steps to follow using the numeral MCMXCIV as an example:

• Start with M = 1000.
• CM = 900 (because C is less than M, so 1000 – 100).
• XC = 90 (because X is less than C, so 100 – 10).
• IV = 4 (because I is less than V, so 5 – 1).

Adding all these values together:

1000 (M) + 900 (CM) + 90 (XC) + 4 (IV) = 1994.

Hence, MCMXCIV converts to 1994.

These rules and guidelines allow you to accurately convert any Roman numeral to its numerical counterpart.

A Step-by-Step Guide to Converting Them into Numbers

Step 1: Assigning Numerical Values to Roman Symbols:

Each Roman numeral symbol corresponds to a specific numerical value. Here’s a quick reference:

• I = 1
• V = 5
• X = 10
• L = 50
• C = 100
• D = 500
• M = 1000

Step 2: Identifying Symbols and Their Values:

Begin by identifying each Roman numeral symbol in the given sequence and determining its numerical value.

Example: Consider the Roman numeral “XIV”:

X = 10

I = 1

V = 5

Step 3: Calculating Total Value:

Add the numerical values of all the symbols together to find the total value.

Example: Using the Roman numeral “XIV”:

X (10) + I (1) + V (5) = 10 + 1 + 5 = 16

Step 4: Handling Subtractive Notation:

Pay attention to instances of subtractive notation, where a symbol of lesser value appears before a symbol of greater value. In such cases, subtract the value of the lesser symbol from the value of the greater symbol.

Example: Let’s convert the Roman numeral “IX”:

IX represents 10 (X) minus 1 (I), which equals 9.

Step 5: Subtracting Values:

Subtract the values of the symbols according to the subtractive notation rules.

Example: Consider the Roman numeral “CM”:

CM represents 1000 (M) minus 100 (C), resulting in 900.

Step 6: Combining Values:

Combine the values of all symbols to determine the overall numerical value of the Roman numeral.

Example: Let’s convert the Roman numeral “MCMLXXXIV”:

M (1000) + CM (900) + L (50) + XXX (30) + IV (4) = 1000 + 900 + 50 + 30 + 4 = 1984

Step 7: Handling Complex Numerals:

For longer Roman numerals with multiple symbols, repeat the steps of identifying, calculating, and combining values until you reach the final total.

Example: Converting the Roman numeral “MMCDXLVII”:

MM (1000 + 1000 = 2000) + CD (500 – 100 = 400) + XL (50 – 10 = 40) + VII (5 + 1 + 1 = 7) = 2000 + 400 + 40 + 7 = 2447

Part6. Numbers to Roman Numerals

The use of Roman numerals persists in modern contexts, such as labeling positions, indicating class rankings, and marking dates on monuments and in media. The conversion of Roman numerals to numbers involves certain rules that help in accurately determining their values. Here are the rules simplified:

Rule 1: Addition

Description: If one or more symbols of lesser value are placed after a symbol of greater value, add the amounts.

Examples:

• VII = 7 (V + II = 5 + 2 = 7)
• LXXX = 80 (L + XXX = 50 + 30 = 80)
• MCCC = 1300 (M + CCC = 1000 + 300 = 1300)

Rule 2: Subtraction

Description: If a symbol is placed before another symbol of greater value, subtract the amount of the smaller symbol from the larger.

Examples:

• CM = 900 (M – C = 1000 – 100 = 900)
• IX = 9 (X – I = 10 – 1 = 9)
• XC = 90 (C – X = 100 – 10 = 90)

Rule 3: Use of Overbars

Description: An overbar on a Roman numeral increases its value by 1,000 times. This is used for representing large numbers.

Examples using Overbars for Larger Values:

• V̅ = 5,000
• X̅ = 10,000
• L̅ = 50,000
• C̅ = 100,000
• D̅ = 500,000
• M̅ = 1,000,000

These rules are crucial for converting Roman numerals into their corresponding numeric values accurately.

Part7. Tips to Remember Roman Numerals

Learn Roman numerals using the following tips and strategies.

Remembering Roman numerals and their rules can be tricky, but with the right strategies, it becomes much easier. Here are some effective tips to help you memorize and understand Roman numerals:

1. Learn the Basic Symbols

Memorize the seven basic symbols that make up all Roman numerals:

• I = 1
• V = 5
• X = 10
• L = 50
• C = 100
• D = 500
• M = 1000

2. Understand the Additive and Subtractive Principles

• Additive Principle: If a smaller or equal value follows another numeral, add its value.
• Subtractive Principle: If a smaller value precedes a larger value, subtract the smaller from the larger.

3. Practice with Common Examples

Regularly practice both reading and writing Roman numerals with familiar numbers. Examples include:

• Clock faces (typically use Roman numerals up to XII)
• Movie series (like Star Wars, Rocky)
• Super Bowls (e.g., Super Bowl LIV)
• Book chapters and sections

4. Use Mnemonics for Subtractive Cases

Create mnemonics for the six subtractive combinations to remember them better:

• IV (4)
• IX (9)
• XL (40)
• XC (90)
• CD (400)
• CM (900)

Example: “I Value Xylophones Like Cows Dig Milk” can be used to remember IV, IX, XL, XC, CD, CM.

5. Group Similar Numerals Together

Understand how numbers cluster around certain base values:

• Numbers around 5: IV (4) and VI (6)
• Numbers around 10: IX (9) and XI (11)
• Numbers around 50: XLIX (49) and LI (51)

Part8. Roman Numerals Solved Examples

To have a better grasp of Roman numerals and their applications, it is essential to understand how to use Roman letters to form numerals. Let’s look at thorough solutions for each of the above examples, including step-by-step reasoning to explain the conversion process:

Detailed Solutions for Roman Numerals

Example 1: Reading Roman Numerals

Roman Numeral: XII

Step-by-Step Solution:

• X = 10
• I = 1
• I = 1

Process: Since the numerals increase or stay the same from left to right, you simply add them up.

Total: 10 + 1 + 1 = 12

Example 2: Writing Roman Numerals

Number: 16

Step-by-Step Solution:

• The closest highest base numeral is X for 10.
• Remaining number is 6, which is written as VI.

Process: Combine these to form XVI.

Roman Numeral: XVI

Advanced Examples Involving Subtractive Notation

Example 3: Reading Roman Numerals

Roman Numeral: CM

Step-by-Step Solution:

• C = 100
• M = 1000

Process: C precedes M, indicating subtraction (as C is less than M).

Result: 1000 – 100 = 900

Example 4: Writing Roman Numerals

Number: 44

Step-by-Step Solution:

• XL represents 40 (as 50 – 10 = 40).
• IV represents 4 (as 5 – 1 = 4).

Process: Combine these to represent 44.

Roman Numeral: XLIV

Combining Multiple Rules

Example 5: Reading Roman Numerals

Roman Numeral: MCMXCIX

Step-by-Step Solution:

• M = 1000
• CM = 900 (1000 – 100)
• XC = 90 (100 – 10)
• IX = 9 (10 – 1)

Process: Add all values together since the numeral pairs are in valid subtractive notation.

Total: 1000 + 900 + 90 + 9 = 1999

Example 6: Writing Roman Numerals

Number: 2023

Step-by-Step Solution:

• MM for 2000
• XX for 20
• III for 3

Process: Combine these values sequentially.

Roman Numeral: MMXXIII

Using Overbars for Large Numbers

Example 7: Reading Roman Numerals with Overbars

Roman Numeral with Overbars: V̅

Step-by-Step Solution:

• V̅ represents 5 multiplied by 1000 due to the overbar.

Result: 5,000

Example 8: Writing Roman Numerals with Overbars0.

Number: 50,000

Step-by-Step Solution:

• L represents 5
• The overbar multiplies the base value (50) by 1000.

Roman Numeral: L̅

Practical Application: Dating and Historical Events

Example 9: Reading Roman Numerals in Context

Roman Numeral on a Monument: MCMXLV

Step-by-Step Solution:

• M = 1000
• CM = 900
• XL = 40
• V = 5

Process: Add all values sequentially, noting that CM and XL are valid subtractive combinations.

Total: 1945

Example 10: Writing Roman Numerals for Current Year

Number (Current Year): 2023

Step-by-Step Solution:

• MM for 2000
• XX for 20
• III for 3

Process: Sequential addition of values.

Roman Numeral: MMXXIII

Understanding these examples thoroughly elucidates how Roman numerals function, from basic operations to complex historical contexts, enhancing both reading and writing skills.

Part9. Practice Questions on Roman Numerals

Certainly! Here are the practice questions on Roman numerals reformulated as multiple-choice questions. Each question includes four options, one of which is the correct answer.

FAQs About Roman Numerals

Q1. Why were Roman numerals invented?

Roman numerals were developed by the ancient Romans as a numeral system to facilitate counting and record-keeping in their society. They were used extensively in the Roman Empire for various purposes, including commerce, administration, and monument inscriptions.

Q2. Can Roman numerals represent large numbers?

Yes, Roman numerals can represent large numbers by combining symbols to create higher values. For instance, “M” represents 1000, and additional symbols such as “V” (5) or “X” (10) can be added to create larger values like 5000 (V with a line above it) or 10000 (X with a line above it).

Q3. Are there any alternatives to Roman numerals in contemporary usage?

Yes, in modern contexts, Arabic numerals (the familiar 0-9 digits) are predominantly used for mathematical calculations and everyday counting due to their simplicity and efficiency. However, Roman numerals continue to hold symbolic and traditional value in certain settings.

Conclusion:

In this article, we explored the fascinating world of “roman numerals” and how understanding them can enhance math skills. We discovered the WuKong Math Advanced Course, offering personalized guidance and engaging math lessons. From basic concepts to complex problem-solving, this course empowers students of all ages to excel in math. By enrolling in the WuKong Math Advanced Course, learners embark on a transformative journey of mathematical discovery. With innovative teaching methods and interactive resources, students develop a deep understanding and love for math. Don’t miss out on this opportunity to unlock your child’s potential in math—enroll them today in the WuKong Math Advanced Course!

Delvair holds a degree in Physics from the Federal University of Maranhão, Brazil. With over six years of experience, she specializes in teaching mathematics, with a particular emphasis on Math Kangaroo competitions. She firmly believes that education is the cornerstone of society’s future. Additionally, she holds the conviction that every child can learn given the right environment and guidance. In her spare time, she enjoys singing and tending to her plants.