Point Slope Form: A Comprehensive Guide (Equation, Calculators & Examples​)

Linear equations form the backbone of algebraic thinking, and among their various representations, point slope form stands out as a particularly powerful tool. Whether you’re a student grappling with the basics of algebra, an educator looking to explain these concepts more effectively, or someone who needs to apply linear equations in real-world scenarios, understanding point slope form is crucial.

This comprehensive guide will cover everything you need to know about point slope form, from its basic definition to practical applications, from point slope form calculations with steps to the most useful digital tools for working with these equations.

What is Point Slope Form?

Point slope form is a fundamental way to write linear equations emphasizing the relationship between any point on a line and the line’s slope. Unlike other forms of linear equations, point slope form is particularly intuitive because it directly relates to how we think about lines – starting at a point and moving with a certain steepness (slope).

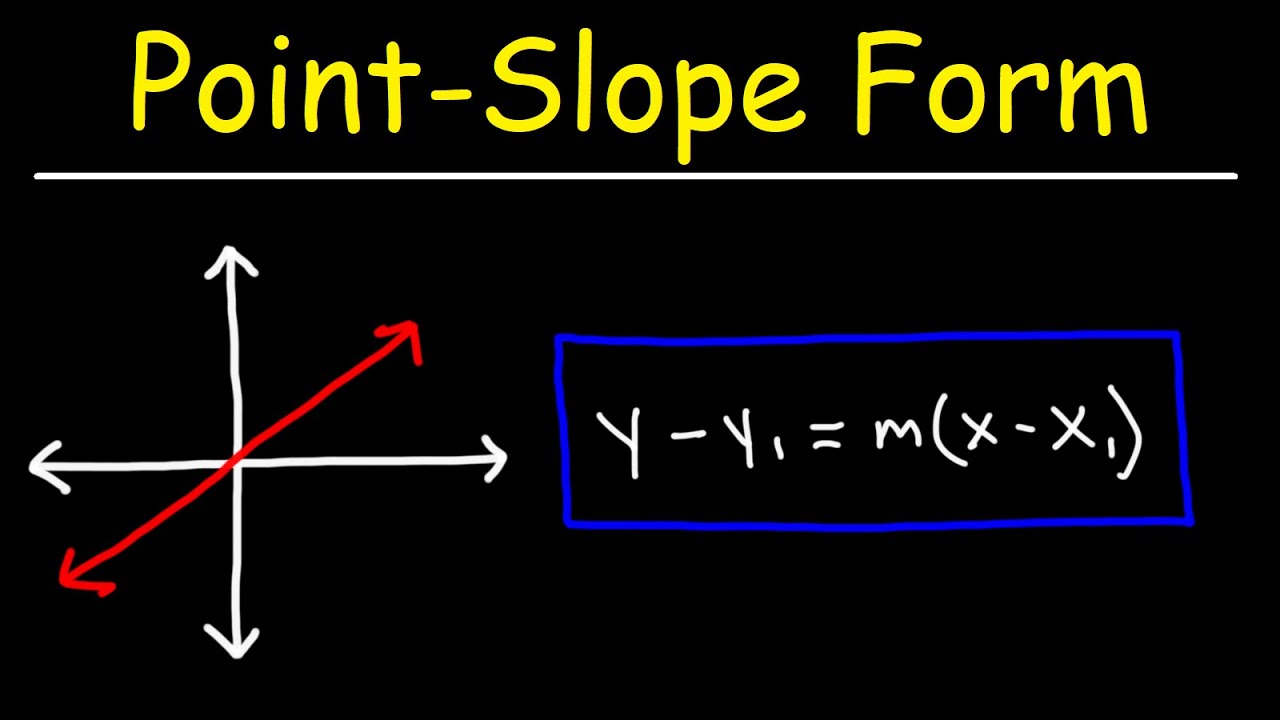
For example, imagine you’re hiking up a mountain trail. If you know your starting point (say, at 1000 feet elevation) and how steep the trail is (rising 300 feet for every 1000 feet of horizontal distance), you can describe any point along that trail. This is exactly what point slope form does mathematically.

Point Slope Form Formula

The point slope form formula is:

y−y1=m(x−x1)

Where:

• (x1​,y1​) is any point on the line
• $m$ is the slope of the line
• (x,y) represents any point on the line

This formula essentially tells us how far any point (x,y) on the line is from our known point (x1,y1), using the slope mmm to measure this relationship.

Point Slope Equation

Let’s see how this formula transforms into an equation. Suppose we have a point (2,3) and a slope of 4. Plugging these values into our formula:

y−3=4(x−2)

To expand this:

1. Distribute the slope:y−3=4x−8
2. Add 3 to both sides: y=4x−5

This final form shows us how any y-coordinate relates to any x-coordinate on our line.

Point Slope Form Calculator

While calculating point slope form by hand is valuable for learning, several digital tools can help verify work or handle more complex calculations. Modern graphing calculators like the TI-84 Plus can quickly generate these equations. Online platforms like Desmos and GeoGebra offer interactive visualizations that help students understand how changing the point or slope affects the line.

How is Using Point Slope Form Helpful When Writing Equations?

Point slope form shines in several scenarios:

• When given a point and slope from real-world data
• When working with parallel or perpendicular lines (since slopes are easily comparable)
• When analyzing rates of change from a specific starting point
• In calculus, when working with tangent lines to curves

This form is particularly useful in physics when describing motion from a starting position or in economics when analyzing price changes from a reference point.

How to Find The Equation of a Line with Slope and Coordinates of a Point?

Finding the equation of a line when given a slope and a point is a fundamental skill in algebra that connects several key mathematical concepts. This method uses the point-slope form of a linear equation, which allows us to construct the precise mathematical relationship between x and y coordinates along the line.

3 Steps to Finding Point Slope Form Equation

Let’s solve this step-by-step using the point (−1,2) and slope m=3:

1. Identify your values:
   • Point: x1=−1,y1=2
   • Slope: m=3
     
2. Plug these values into the point slope formula: y−2=3(x−(−1))
3. Simplify: y−2=3(x+1)y – 2 = 3x + 3y=3x+5

What’s the Difference Between Slope Intercept Form and Point Slope Form?

The Slope-Intercept Form and the Point-Slope Form are both ways to represent the equation of a straight line, but they highlight different aspects of the line. Here’s a breakdown of their differences:

1. Slope-Intercept Form

The Slope-Intercept Form is written as:

y=mx+b

Where:

• m is the slope of the line (the rate of change of $y$ with respect to x),
• b is the y-intercept (the point where the line crosses the y-axis, i.e., when x=0).

Key Features:

• Focuses on slope and y-intercept.
• It’s useful when you know the slope and the y-intercept directly, or when you’re graphing a line starting from the y-axis.
• The equation is easy to use for quickly graphing a line, as you just need the slope and the y-intercept to plot it.

Example:

If the equation is y=2x+3, the slope is 2, and the y-intercept is 3 (so the line crosses the y-axis at (0, 3)).

2. Point-Slope Form

The Point-Slope Form is written as:

y−y1=m(x−x1)

Where:

• $m$ is the slope of the line,
• (x1,y1) is a specific point on the line (any point that lies on the line).

Key Features:

• Focuses on a point and the slope.
• It’s useful when you know the slope of the line and a specific point on the line.
• This form is particularly handy when you’re given a point on the line and the slope, and you need to write the equation of the line.

Example:

If you know the slope m=2 and the point (3,4) on the line, the equation in point-slope form would be:

y−4=2(x−3)

Summary of Differences:

Aspect	Slope-Intercept Form (y = mx + b)	Point-Slope Form (y – y₁ = m(x – x₁))
Focus	Slope and y-intercept	Slope and a point on the line
Best for	When slope and y-intercept are known	When the slope and a specific point are known
Form	y=mx+b	y−y1=m(x−x1)
Uses	Easy for graphing when y-intercept is given	Ideal for writing equations from a point and slope
Example	y=2x+3	y−4=2(x−3)

Converting Between the Forms:

• From Point-Slope to Slope-Intercept: If you have the point-slope form y−y1=m(x−x1), you can simplify it into slope-intercept form by expanding and solving for y.
• From Slope-Intercept to Point-Slope: If you know the slope and y-intercept, you can easily write the equation in point-slope form by using any point on the line (like (0,b)).

Both forms are powerful and help in different situations, depending on your information about the line!

Conclusion

Point slope form represents a powerful tool in mathematics, bridging the gap between abstract concepts and practical applications. Its strength lies in its intuitive connection to how we naturally think about lines – starting somewhere and moving with a consistent slope. Whether you’re studying calculus, physics, or real-world data analysis, understanding point slope form provides a solid foundation for mathematical problem-solving.

FAQs about Point Slope Form

Q1: Why use point slope form instead of slope intercept form?

Point slope form is particularly useful when you’re given a specific point and slope, eliminating the need to calculate the y-intercept. It’s also more intuitive for many real-world applications.

Q2: Can point slope form be used for vertical lines?

Vertical lines have undefined slopes and cannot be written in point-slope form. These lines are typically written as x=a, where a is the x-coordinate.

Q3: How do you convert from point slope to slope intercept form?

Simply distribute the slope term and solve for y. For example, y−2=3(x−1) becomes y=3x−1 after distribution and algebra.

Q4: Can you use any point on the line for point slope form?

Yes! Any point on the line will give you an equivalent equation, though the initial form might look different before simplification.