Rational Numbers: Definition, Examples & Key Properties

Rational numbers are one of the most important concepts in mathematics, acting as the foundation for many other branches of number theory, algebra, and beyond. They are versatile, widely used in different fields, and provide the foundation for understanding the relationship between integers and fractions. A few rational numbers can be listed as 3, 4.57, 3/4, 0, -7, and so on.

In this article, we will dive into the definition of rational numbers, explore their key properties, and walk through various examples to help you better understand them. We will also discuss how rational numbers differ from irrational numbers and how to work with them in various mathematical operations.

What is a Rational Number?

Rational numbers are numbers that can be expressed as the ratio of two integers, specifically in the form p/q, where p is the numerator (the top number) and q is the denominator (the bottom number), and q ≠ 0. The term rational comes from the word “ratio,” which reflects the fact that rational numbers are always the ratio of two integers.

These numbers include integers, proper fractions, improper fractions, and mixed fractions, and they can be either positive or negative. Rational numbers can also be represented in decimal form, where they may either terminate (end after a certain number of digits) or repeat (the digits follow a regular pattern).

In the following sections, we’ll explore the key properties of rational numbers, types of rational numbers, and provide examples to help clarify the concept.

Key Properties of Rational Numbers

Rational numbers have several important properties that make them distinct from other types of numbers. For example, a rational number can be expressed as the ratio of two integers, where the denominator is a non-zero integer. Let’s take a closer look at some of the key properties of rational numbers:

Expressed as a Fraction

A rational number is any number that can be expressed as the ratio of two integers, and this includes both positive and negative numbers. A rational number’s denominator (the bottom number of the fraction) cannot be zero. Division by zero is undefined, which means any fraction with q = 0 is not a rational number.

Decimal Form

When rational numbers are written in decimal form, they can either be terminating decimals (ending after a certain number of digits) or repeating decimals (where the digits repeat in a pattern). For example, 0.75 is a terminating decimal, where the digits stop after the decimal point. On the other hand, 0.333… (where the digit 3 repeats indefinitely) is a repeating decimal, and the repeating pattern after the decimal point is 3.

Closure Property

Rational numbers are closed under addition, subtraction, multiplication, and division (except division by zero). This means that performing any of these operations on rational numbers will always result in another rational number. For example, adding 2/3 and 3/5 will give you a rational number.

Negative and Positive Numbers

Rational numbers can be both positive and negative. Negative rational numbers are simply those where either the numerator or the denominator (but not both) is negative. For example, -2/3 and 3/-4 are both negative rational numbers.

Equivalent Fractions

Two fractions are equivalent if they represent the same value. For example, 1/2 is equivalent to 2/4, because they both represent the same ratio.

Types of Rational Numbers

Rational numbers are a broad category of numbers that can be expressed as the ratio of two integers, where the denominator is not zero. These numbers can take various forms, each with distinct characteristics. Let’s explore the different types of rational numbers:

1. Integers

Integers are the simplest form of rational numbers. They include all positive whole numbers, zero, and negative whole numbers. Integers can always be expressed as a ratio of two integers, with the denominator being 1.

For example:

• 5 can be written as 5/1.
• -3 can be written as -3/1.
• 0 can be written as 0/1.

Since these numbers have a denominator of 1, they are classified as rational numbers.

2. Proper Fractions

A proper fraction is a rational number where the numerator is smaller than the denominator. The value of a proper fraction is always less than 1.

For instance:

• 2/5 is a proper fraction because the numerator (2) is smaller than the denominator (5).
• -3/8 is also a proper fraction because the numerator (-3) is smaller than the denominator (8).

Proper fractions are rational because they represent a ratio of two integers, with a non-zero denominator.

3. Improper Fractions

An improper fraction is a rational number where the numerator is greater than or equal to the denominator. The value of an improper fraction is either equal to or greater than 1.

For example:

• 7/4 is an improper fraction because the numerator (7) is greater than the denominator (4).
• 5/5 is also an improper fraction because the numerator (5) equals the denominator (5).

Improper fractions can often be converted into mixed fractions or decimals, but they are still rational numbers because they can be expressed as a ratio of two integers.

4. Terminating Decimals

A terminating decimal is a decimal that comes to an end after a finite number of digits. All terminating decimals are rational because they can be written as fractions with integers in the numerator and denominator.

For example:

• 0.75 is a terminating decimal, which is equivalent to the fraction 3/4.
• -2.5 is a terminating decimal, which is equivalent to the fraction -5/2.

Terminating decimals occur when the denominator of the fraction (in its lowest terms) is a power of 2, 5, or both. For example, 1/8 gives 0.125, which is a terminating decimal.

5. Repeating Decimals

A repeating decimal is a decimal in which one or more digits repeat infinitely. These decimals are rational numbers because they can be expressed as fractions.

For example:

• 0.333… (where 3 repeats) is equivalent to the fraction 1/3.
• 0.666… (where 6 repeats) is equivalent to the fraction 2/3.

Such decimals occur when the denominator of a fraction (in its simplest form) has prime factors other than 2 and 5. For instance, 1/3 gives the decimal 0.333…, which can be written as a ratio of two integers.

6. Negative Rational Numbers

Negative rational numbers are those where either the numerator, the denominator, or both are negative. These numbers can appear as negative integers, fractions, or decimals. Essentially, they are rational numbers with a negative value.

For example:

• -5/7 is a negative rational number because the numerator is negative.
• -1.5 is a negative rational number because it can be written as -3/2.

Negative rational numbers behave the same way as positive rational numbers in arithmetic operations, with the additional consideration of signs.

How to identify rational numbers?

Rational numbers come in many forms whether positive or negative, rational numbers can always be expressed as a fraction of two integers.

This flexibility allows rational numbers to be a versatile tool in mathematics, appearing in everything from basic arithmetic to advanced number theory.

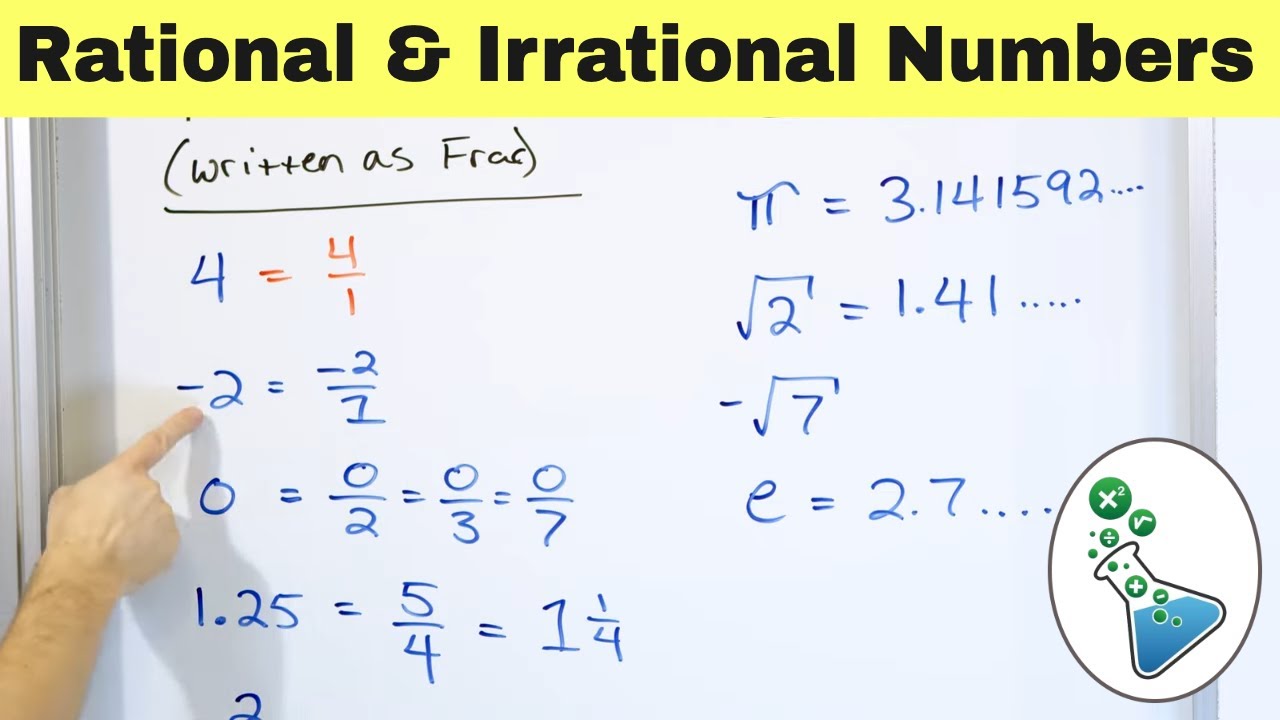
Recognizing the different types of rational numbers is an essential skill in understanding and manipulating numbers in everyday life and mathematical problem-solving.

Let’s look at a few ways to identify rational numbers:

• Fractions: Any number expressed as a simple fraction with integers as the numerator and denominator is a rational number. For instance, 3/4, -5/6, and 2/3 are all rational numbers.
• Integers: Every integer is a rational number because it can be written as a fraction. For example, the number 7 can be written as 7/1, which is a rational number.
• Terminating Decimals: If a decimal number has a finite number of digits, it is a rational number. For example, 0.5 and 2.75 are rational numbers because they have terminating decimal representations.
• Repeating Decimals: If a decimal number has a repeating pattern, it is also a rational number. For example, 0.333… is rational because it repeats indefinitely, and it can be written as 1/3.

Adding and Subtracting Rational Numbers

Working with rational numbers often involves basic arithmetic operations like addition and subtraction. To add or subtract rational numbers, follow these steps:

1. Find a Common Denominator:
   If the fractions have different denominators, first find a common denominator. For example, to add 2/3 and 3/5, the common denominator is 15.
2. Rewrite the Fractions:
   Rewrite each fraction with the common denominator. For example, 2/3 becomes 10/15, and 3/5 becomes 9/15.
3. Add or Subtract the Numerators:
   After rewriting the fractions with the same denominator, simply add or subtract the numerators. In this case, 10/15 + 9/15 = 19/15.
4. Simplify if Necessary:
   If the resulting fraction can be simplified, do so. For example, 2/4 can be simplified to 1/2.

Multiplying and Dividing Rational Numbers

Multiplying and dividing rational numbers is straightforward. Here’s how to do it:

• Multiplication: Multiply the numerators and multiply the denominators. For example, multiplying 2/3 by 3/5 results in 6/15, which can be simplified to 2/5.
• Division: To divide rational numbers, multiply by the reciprocal of the divisor. For example, dividing 2/3 by 4/5 involves multiplying 2/3 by 5/4, giving a result of 10/12, which simplifies to 5/6.

Rational Numbers vs. Irrational Numbers

While rational numbers are numbers that can be expressed as the ratio of two integers, irrational numbers cannot. Irrational numbers include numbers like √2, π, and e, which cannot be written as a fraction of two integers.

Unlike rational numbers, irrational numbers have non-terminating and non-repeating decimal expansions.

Definition of Irrational Numbers

Irrational numbers are real numbers that cannot be expressed as p/q, where p and q are integers. These numbers have decimal expansions that never terminate or repeat in a regular pattern.

Difference Between Rational and Irrational Numbers

The primary difference between rational and irrational numbers is that rational numbers can be written as fractions, whereas irrational numbers can not.

• Rational Numbers: Can be written as p/q, where p and q are integers, with q ≠ 0. Their decimal expansions are either terminating or repeating.
• Irrational Numbers: Cannot be written as p/q, and their decimal expansions never terminate or repeat. Examples include π and √2.

Practice Problems

To deepen your understanding of rational numbers, try solving the following practice problems. Each one is designed to test your ability to identify, simplify, and work with rational numbers in various forms. The solutions will help you grasp the concepts more clearly.

Problem 1: Identifying Rational Numbers

Which of the following numbers are rational?

A) 0.75
B) √5
C) -3
D) π
E) 1/2

Hint: A rational number can be written as a fraction of two integers.

Problem 2: Writing Decimals as Fractions

Convert the following repeating decimal into a fraction:
0.666…

Hint: Recognize that 0.666… is a repeating decimal and can be written as 2/3.

Problem 3: Simplifying Fractions

Simplify the following fractions:
A) 12/18
B) 45/60
C) 100/400

Hint: Find the greatest common divisor (GCD) of the numerator and denominator and divide both by it.

Problem 4: Adding Rational Numbers

Add the following rational numbers:
A) 2/5 + 3/10
B) -4/9 + 7/9
C) -1/3 + 2/5

Hint: For adding fractions, make sure the denominators are the same, or find a common denominator first.

Problem 5: Subtracting Rational Numbers

Subtract the following rational numbers:
A) 5/8 – 3/4
B) -7/12 – 5/6
C) 1/2 – 2/3

Hint: Remember that subtracting fractions requires finding a common denominator, then subtracting the numerators.

Solutions:

Here are the solutions for you to check your answers:

1.

• A) 0.75 is rational (can be written as 3/4).
• B) √5 is irrational (it cannot be written as a fraction of two integers).
• C) -3 is rational (it can be written as -3/1).
• D) π is irrational (it cannot be expressed as a fraction).
• E) 1/2 is rational (it’s already in fraction form).

2.

• 0.666… = 2/3

3.

• A) 12/18 simplifies to 2/3.
• B) 45/60 simplifies to 3/4.
• C) 100/400 simplifies to 1/4.

4.

• A) 2/5 + 3/10 = 7/10
• B) -4/9 + 7/9 = 3/9 = 1/3
• C) -1/3 + 2/5 = 7/15

5.

• A) 5/8 – 3/4 = -1/8
• B) -7/12 – 5/6 = -17/12
• C) 1/2 – 2/3 = -1/6

Conclusion

Rational numbers are a fundamental concept in mathematics, encompassing all numbers that can be expressed as the ratio of two integers. From simple fractions to repeating and terminating decimals, rational numbers have unique properties that distinguish them from irrational numbers.

Understanding rational numbers is essential for solving various mathematical problems and for grasping the relationships between different types of numbers.

FAQs about Rational Numbers

Q1: What is a rational number example?

A rational number is any number that can be expressed as the ratio of two integers, where the denominator is not zero.

An example of a rational number is 3/4, which is the ratio of the integers 3 (numerator) and 4 (denominator). Other examples include -5/2 and 7.

Q2: Is 3.14 a rational number?

Yes, 3.14 is a rational number. It can be written as the fraction 314/100, where both the numerator and denominator are integers. Therefore, 3.14 is a rational number because it can be expressed as a ratio of two integers.

Q3: Is 0.777 a rational number?

Yes, 0.777 is a rational number. It is a repeating decimal (0.777…) and can be written as the fraction 7/9, which is the ratio of two integers. Repeating decimals are always rational numbers.

Q4: Is 7 a rational number?

Yes, 7 is a rational number. It can be expressed as the fraction 7/1, where both 7 and 1 are integers, and the denominator is not zero. Hence, 7 is a rational number.